Solution-CF102920L Two Buildings【分治】【决策单调性】

优秀的分治题目。是“2020-2021 ACM-ICPC, Asia Seoul Regional Contest”的一道题。

Description

There are $n$ buildings along a horizontal street. The buildings are next to each other along the street, and the $i$-th building from left to right has width $1$ and height $h_i$. Among the $n$ buildings, we are to find two buildings, say the $i$-th building and $j$-th building with $i<j$, such that $(h_i+h_j)*(j−i)$ is maximized.

For example, the right figure shows $5$ buildings, with heights $1$, $3$, $2$, $5$, $4$, from left to right. If we choose the first $2$ buildings, then we get $(1+3)*(2−1)=4$. If we choose the first and fifth buildings, then we $(1+4)*(5−1)=20$. The maximum value is achieved by the second and fifth buildings with heights $3$ and $4$, respectively: $(3+4)*(5−2)=21$.

Write a program that, given a sequence of building heights, prints $\max_{1\le i<j\le n}(h_i+h_j)*(j−i)$.

Input

Your program is to read from standard input. The input starts with a line containing an integer $n$ ($2\le n\le 1,000,000$), where $n$ is the number of buildings. The buildings are numbered $1$ to $n$ from left to right. The second line contains the heights of $n$ buildings separated by a space such that the $i$-th number is the height $h_i$ of the $i$-th building ($1\le h_i\le 1,000,000$).

Output

Your program is to write to standard output. Print exactly one line. The line should contain $\max_{1\le i<j\le n}(h_i+h_j)*(j−i)$.

Examples

input

5
1 3 2 5 4

output

21

input

5
8 3 6 3 1

output

36

题意：

有 $n(2\le n\le 1000000)$ 栋楼房，第 $i$ 栋高度为 $h_i(1\le h_1\le 1000000)$。问两栋楼房所能构成的 $(h_i+h_j)*(j−i)$ 最大值是多少。

题解：

为了方便，我们把要求的式子转化为 $(h_i-(-h_j))\times(j-i)$，这样构造出数列 $-h_i$，就是坐标系中 $x$ 轴上方和 $x$ 轴下方两个数列中选出两个 $h$ 进行计算。

如果存在 $i<j$，同时 $-h_i<-h_j$，那么 $j$ 一定比 $i$ 更优。可以用单调栈来把数列 ${h_i}$ 和 ${-h_i}$ 都变为单调的。得到单增的 ${H_i}$ 和 ${-H_i}$。

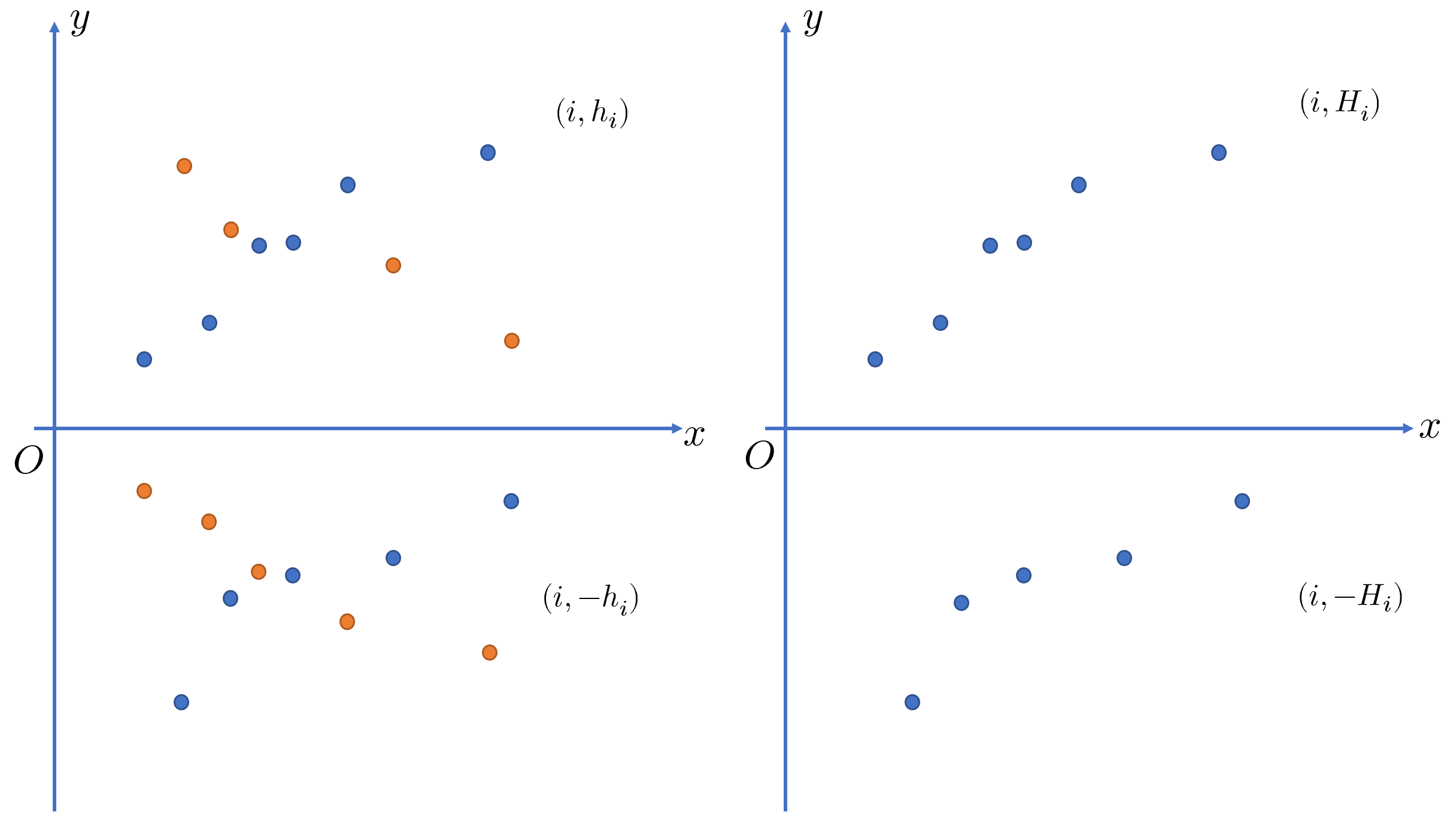
如图，图中橙色的点删除后，对答案产生贡献的点只会在蓝色中产生。

而蓝色的点 ${H_i}$ 和 ${-H_i}$ 对应了两段递增的序列（此时下标 $i$ 不一定连续）。最终答案为 $[H_i-(-H_j)]\times(j-i)$。假设对于选定的 $H_i$，对应 $-H_j$ 为最优解，即 $\forall k>j,[H_i-(-H_j)]\times(j-i)\geqslant[H_i-(-H_k)]\times(k-i)$，可知

$$\begin{aligned}
[H_i-(-H_j)]\times(j-i)&\geqslant[H_i-(-H_k)]\times(k-i)\\ [H_i-(-H_j)]\times(j-i)\color{red}{-[H_i-(-H_k)]\times(j-i)}&\geqslant[H_i-(-H_k)]\times(k-i)\color{red}{-[H_i-(-H_k)]\times(j-i)}\\ [(-H_k)-(-H_j)]\times(j-i)&\geqslant[H_i-(-H_k)]\times(k-j) \end{aligned}$$

因此对于 $l<i$，对上式进行放缩，得

$$\begin{aligned}\\ [(-H_k)-(-H_j)]\times(j-i)&\geqslant[H_i-(-H_k)]\times(k-j)\\ [(-H_k)-(-H_j)]\times(j-i)\color{blue}{+[(-H_k)-(-H_j)]\times(i-l)}&\geqslant[H_i-(-H_k)]\times(k-j)\color{red}{-(H_i-H_l)\times(k-j)}\\ [(-H_k)-(-H_j)]\times(j-l)&\geqslant[H_l-(-H_k)]\times(k-j)\\ [(-H_k)-(-H_j)]\times(j-l)\color{blue}{+[H_l-(-H_k)]\times(j-l)}&\geqslant[H_l-(-H_k)]\times(k-j)\color{blue}{+[H_l-(-H_k)]\times(j-l)}\\ [H_l-(-H_j)]\times(j-l)&\geqslant[H_l-(-H_k)]\times(k-l) \end{aligned}$$

所以当 $H_i$ 对应的最优解位置为 $-H_j$ 时，$l<i$ 的 $H_l$ 对应的最优解位置 $-H_k$ 满足 $k\geqslant j$。

如果上式推导过于复杂，它对应的图形推导如下。

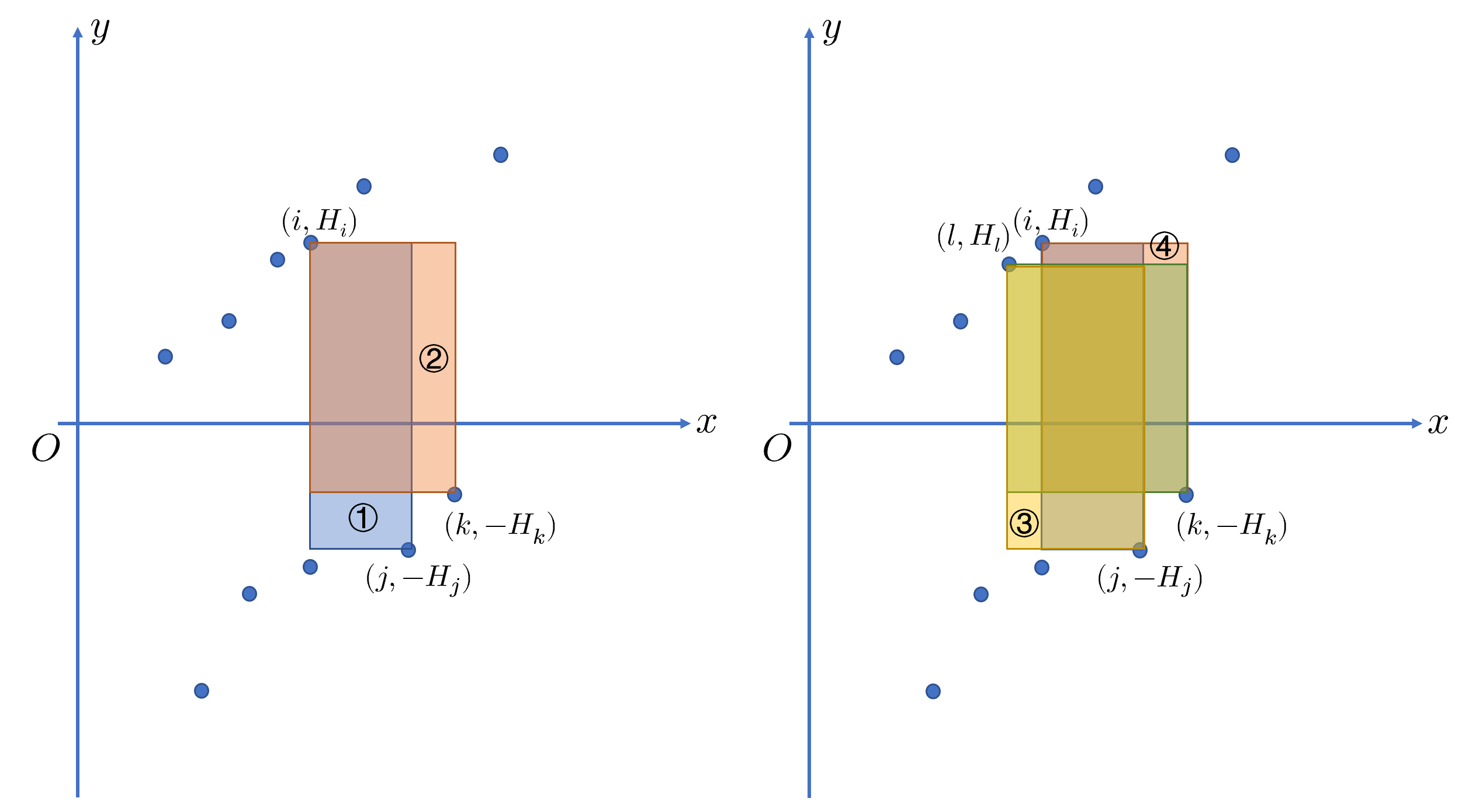
左图中，如果 $H_i$ 对应的最优解是 $H_j$，那么①的面积 $S_1$ 一定比②的 $S_2$ 大。右图中，那么对 $l<i$，已知 $S_1\geqslant S_2$，有 $S_1+S_3\geqslant S_2-S_4$，所以 $l$ 对应的最优解一定不会在 $j$ 右边。

此时再考虑上边的推导，便能清楚理解了。

分治递归，时间复杂度为 $O(n\log n)$。

Code：

#include<cstdio>
#include<cstring>
#include<iostream>
#include<algorithm>
#include<cmath> 
using namespace std;
#define ll long long
int a[1001000],b[1001000],c[1001000];
int bp[1001000],cp[1001000];
ll ans=0;
void solve(int l,int r,int L,int R)
{
	if(l>r)
		return;
	int mid=(l+r)>>1,q=0;
	ll Ans=0;
	for(int i=L;i<=R;++i)
	{
		if(Ans<1ll*(cp[i]-bp[mid])*(b[mid]+c[i]))
		{
			q=i;
			Ans=1ll*(cp[i]-bp[mid])*(b[mid]+c[i]);
		}
	}
	ans=ans>Ans?ans:Ans;
	solve(l,mid-1,L,q);
	solve(mid+1,r,q,R);
}
int main() 
{
	int n;
	scanf("%d",&n);
	for(int i=1;i<=n;++i)
		scanf("%d",&a[i]);
	int ii=0,iii=0;
	c[0]=1e8;
	for(int i=1;i<=n;++i)
	{
		if(a[i]>b[ii])
		{
			b[++ii]=a[i];
			bp[ii]=i;
		}
			
		while(a[i]>=c[iii])
			--iii;
		c[++iii]=a[i];
		cp[iii]=i;
	}
	solve(1,ii,1,iii);
	printf("%lld\n",ans);
	return 0;
}